Blog Archives

Thought Experiment 44: Till Death Us Do Part

2/29/2016

Las Vegas' Little White Wedding Chapel - Site of At Least One Rational Wedding (My Own)

The title of this week's thought experiment sounds very promising to me. Especially since yesterday was my own fourteenth wedding anniversary. Let's see what Baggini has cooked up for me to think about as I reflect on my own marriage.

---------------------------------------------------
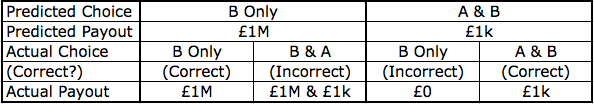
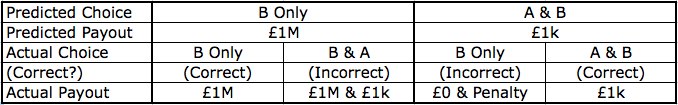
Harry and Sophie wanted to take seriously the words the minister would utter as they exchanged rings: "These two lives are now joined in one unbroken circle." This meant putting their collective interest first, and their individual interests second. If they could do that, the marriage would be better for both of them.
But Harry had seen his own parents divorce and too many friends and relations hurt by betrayal and deceit to accept this unquestioningly. The calculating part of his brain reasoned that, if he put himself second, but Sophie put herself first, Sophie would get a good deal from the marriage but he wouldn't. In other words, he risked being taken for a mug if he romantically failed to protect his own interest.
Sophie had similar thoughts. They had even discussed the problem and agreed that they really would not be egotistical in the marriage. But neither could be sure the other would keep their part of the bargain, so the safest course of action for both was to secretly look out for themselves. That inevitably meant the marriage would not be as good as it could have been. But surely it was the only rational course of action to take?

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 130.
---------------------------------------------------

Oh well. It looks like this is going to be another short post on Friday. I'm starting to recognise the lame thought experiments now as the ones that don't have an outside source attached to them. It's almost as if Baggini needs a partner or something...

5 Comments

Response to Thought Experiment 43: Future Shock

2/26/2016

0 Comments

Can a Republican senator stop a crazed madman??

If you are going to write a book with precisely 100 thought experiments in them, it is unlikely that the history of philosophy has produced exactly such a perfectly round number of ideas to draw from. Either there are many more than 100 out there, in which case you try to choose the best ones for your purpose, or there are fewer than 100 strong and diverse thought experiments out there, in which case you make up a few to round out the marketing premise for your book. This week's thought experiment, along with the slight redundancy already experienced in the past, sure looks like proof of the latter...

---------------------------------------------------
"Drew! I haven't seen you since college, twenty years ago! My God, Drew—what are you doing with that gun?"
"I've come to kill you," said Drew, "just as you asked me to."
   "What the hell are you talking about?"
   "Don't you remember? You said to me, many times, 'If I ever vote Republican, then shoot me.' Well, I just read you're actually a Republican senator. So you see, you must die."
   "Drew, you're crazy! That was twenty years ago! I was young, I was idealistic! You can't hold me to that!"
   "It was no casual, flippant remark, senator. In fact, I have here a piece of paper, signed by you and witnessed by others, instructing me to do this. And before you tell me not to take that seriously, let me remind you that you voted for a bill recently in favour of living wills. In fact, you've got one yourself. Now tell me this: if you think people in the future should carry out your wish to kill you if you get dementia or fall into a permanent vegetative state, why shouldn't I carry out your past wish to kill you if you became a Republican?"
   "I've got an answer to that!" screamed the sweating senator. "Just give me a few minutes!"
   Drew cocked the pistol and aimed. "You'd better be quick."

Baggini, J., The Pig That Wants to Be Eaten, and 99 other thought experiments, 2005, p. 127.
---------------------------------------------------

This is a simple matter of using logic to point out the flaws in Drew's argument. Based on what we're seeing in the current American presidential debate, there's no guarantee the Republican senator will be able to provide that answer, but it's easy for us. As Baggini says in his writeup:

"There is a good answer to Drew's question. ... The key difference here is that [living wills] are there to prepare for the eventuality that no future self will be competent to make a choice. In that situation, the best qualified person to do so may well be the past self rather than a present other. That's the answer the senator should give."

Echoing that, reader atthatmatt commented on Monday:

"So the guy is invoking the written will as having force, but the person who wrote the will is standing in front of him and negating bearing the old written will simply by speaking. ... I don't think wills even exist to force our own hand in the future. They exist to force OTHER people's hands when we can't speak for ourselves."

So, since this is abundantly clear to the original author of the problem, as well as to (at least one of) my intended readers, I won't bore you by belaboring this any further.

I apologize that this post didn't bring up any new philosophical issues; I do try to make all the posts on this blog unique and intriguing. But the fact that this was such a simple problem is actually an interesting result in itself. Given that Julian Baggini is a competent author (and there is much evidence to suggest that he is!), the relative lameness of an entry or two from him means that once I finish these 100 thought experiments, I really will have completed a thorough survey of the topics usually covered by the thought experiment field, and not just some subjective slice of it. Seeing as how I'm always trying to apply the MECE principle to philosophy issues, this shows we're well on our way to a Collectively Exhaustive outcome. Hooray for that!

0 Comments

Thought Experiment 43: Future Shock

2/22/2016

2 Comments

Can any of those me's talk for me now?

Remember all those promises I made when I listened to Rush Limbaugh in college? Yeah, don't hold me to them. Here's why.

---------------------------------------------------
"Drew! I haven't seen you since college, twenty years ago! My God, Drew—what are you doing with that gun?"
"I've come to kill you," said Drew, "just as you asked me to."
   "What the hell are you talking about?"
   "Don't you remember? You said to me, many times, 'If I ever vote Republican, then shoot me.' Well, I just read you're actually a Republican senator. So you see, you must die."
   "Drew, you're crazy! That was twenty years ago! I was young, I was idealistic! You can't hold me to that!"
   "It was no casual, flippant remark, senator. In fact, I have here a piece of paper, signed by you and witnessed by others, instructing me to do this. And before you tell me not to take that seriously, let me remind you that you voted for a bill recently in favour of living wills. In fact, you've got one yourself. Now tell me this: if you think people in the future should carry out your wish to kill you if you get demential or fall into a permanent vegetative state, why shouldn't I carry out your past wish to kill you if you became a Republican?"
   "I've got an answer to that!" screamed the sweating senator. "Just give me a few minutes!"
   Drew cocked the pistol and aimed. "You'd better be quick."

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 127.
---------------------------------------------------

What do you think? Can you come up with a good answer for Drew as to why our future selves should be beholden to our present selves in some situations but not in others? I bet Friday Ed will know. I'm gonna wait for him to show up and tell me why.

2 Comments

Response to Thought Experiment 42: Take the Money and Run

2/19/2016

0 Comments

This week's thought experiment is derived from one that was originally designed to be an unsolvable logic puzzle. In the book I'm drawing these experiments from, author Julian Baggini has added a wrinkle that still leaves it unsolvable, but it does add one more topic for debate. To be honest, this isn't super interesting or morally nuanced like most of the other philosophy thought experiments I've considered. In fact, it's hardly even philosophy in my opinion. But you need good logic skills to do good philosophy, so let's look at this problem, crank through the logic of it, and discuss our options. Some deep rational problem solving is rarely a complete waste of time.

---------------------------------------------------
'Marco the Magnificent will now demonstrate his extraordinary powers of precognition! You, sir! What is your name?'
   'Frank,' replied Frank, to the fairground showman.
   'Frank, I know your future! I know all futures, including those of stocks and shares! Which is why I have the money to give away to you in this demonstration of my powers! Behold, two boxes! One you can see is open. It contains £1,000. The other is closed. It contains either £1 million or nothing at all! You may take either box or both. But be warned! I know how you will choose. If you take just the closed box, it will contain £1 million. If you take both, it will be empty. And if I am wrong, I will give £1 million, which you see before you, to a random member of the crowd!'
   Everyone gasped as Marco opened a suitcase full of £50 notes.
   'Ladies and gentlemen. I have performed this miracle one hundred times and never been wrong, as independent observers have testified. And if you observe the closed box, which is now ten feet from me, you will see that nothing I do can now alter its contents. So, Frank. What will you choose?'

Source: Newcomb's Paradox, devised by William Newcomb and popularised in 'Newcomb's Problem and Two Principles of Choice' by Robert Nozick, in Essays in Honour of Carl G. Hempel (1970).

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 124.
---------------------------------------------------

Why is this a paradox exactly? In case it wasn't clear from your first read through the problem, here's Baggini's explanation of the difficulty:

"It seems clear that [Frank] should choose only the closed box. That way he will get £1 million, instead of just £1,000. But wait. As Frank reaches for the closed box, a thought enters his mind. That box contains £1 million or it doesn't. Nothing he does can change that fact. So if it does contain the money, it is not going to disappear if he also takes the open box. Similarly, if it is empty, £1 million is not going to magically appear in it if he leaves the open box behind. His choice cannot change what is in the closed box. So if he takes the open box or not, the amount in the closed one will remain the same. Therefore he may as well take both, since he can't have less money as a result. Hence we have a paradox. Two lines of reasoning, both seemingly impeccable, lead to contradictory conclusions. One concludes that he should take only the closed box; the other that he may as well take both."

When this problem—known as Newcomb's Paradox—has been discussed in the past, there haven't been any penalties attached for getting the prediction wrong. There were just various versions of who the predictor was (psychic, alien, god, brain scanning computer, etc.) and how certain this predictor was of getting his/her/its prediction right. Without the penalty attached, the permutations for analysis look like this:

Analysis of the problem as originally written.

I should start by mentioning briefly that the choice of boxes in this problem is usually either "B Only" or "A & B". Baggini writes that you may take "either box or both," but there's absolutely no reason to ever choose A by itself. There are no benefits to doing so and no variables or hidden information involved with that choice, so there's no need to analyse it.

So, at the beginning of these initial rules, the clear choice, as Baggini points out, is "B only". If Marco predicts that, Frank's payout is £1,000,000. Once that prediction is set though, then "A & B" becomes the best choice for Frank as his payout would then climb to £1,001,000. Marco would know this, however, so he would predict "A & B", which would drop Frank's payout to £1,000. Frank would know this is worse than where he started though, so he would revert to "B only", which Marco would predict, and we'd be back to the beginning. We're stuck in a loop here because for every choice Frank makes, he can see how it would be better to flip that choice, but since Marco can always foresee that, he keeps flipping his choice as well. Rather than stand there forever without being able to make a decision (which yields £0 while you are waiting), Frank might as well take the perfection of the predictions at face value and choose "B Only" even if £1,000,000 is less than £1,001,000.

Traditionally for this problem, there is also some small doubt expressed about how perfect these predictions are. In that case, you also have to weigh the chance of getting £0 into the mix in the event that your choice of "B Only" was incorrectly predicted. In those variations of this problem, it becomes a question of how certain you need to be of getting £1,000,000 vs. the absolute certainty of getting £1,000. There's some element of that here since we're dealing with a carnival barker backed by "independent observers", but it's no use speculating over the trustworthiness of this scenario as it's really unknowable. The mathematical solution in this case would be to take "B Only" for any certainty over 1% about the accuracy of the prediction (then the expected value of "B Only" > £1M x 1% > £1,000), but as I said, this quantification of certainty is really unknowable.

Now, let's look at what happens given the wrinkle Baggini has added about the £1,000,000 penalty being paid to a random member of the crowd in the event that Marco's prediction is wrong. Plugging that change into the above table, we see:

Analysis of the problem as currently stated.

Once again, we're stuck in the same circular loop. As long as Marco holds to his promise of predicting Frank's choice with 100% accuracy, then for every choice Frank makes, he can see how it would be better to flip that choice, but since Marco can always foresee that, he keeps flipping his choice as well. The wrinkle of the penalty only changes the calculation of the event where Marco's predictions aren't correct, but as I already said above, that calculation is essentially unknowable here.

This logic problem has dragged on farther than I wanted it to, but I hope it has been rigorously explained enough. I could talk about the epistemology of not being able to change the past with a present choice. I could talk about the effect of free will on efforts to predict human behaviour. I could talk about the realm of emotional vs. rational decision making about gains, losses, and missed opportunities. Or I could talk about the math behind Frank making various deals with the audience to share the £1M penalty as a hedge against the downside of choosing "B Only". But by now I hope that you could predict what I would say about any one of those discussions....

0 Comments

Thought Experiment 42: Take the Money and Run

2/15/2016

0 Comments

Which way do we go?

This week's thought experiment is going to delve into the philosophy of decision theory, but it's a bit abstruse so I hope you don't decide to give up on this whole project of mine because of that...

---------------------------------------------------
'Marco the Magnificent will now demonstrate his extraordinary powers of precognition! You, sir! What is your name?'
   'Frank,' replied Frank, to the fairground showman.
   'Frank, I know your future! I know all futures, including those of stocks and shares! Which is why I have the money to give away to you in this demonstration of my powers! Behold, two boxes! One you can see is open. It contains £1,000. The other is closed. It contains either £1 million or nothing at all! You may take either box or both. But be warned! I know how you will choose. If you take just the closed box, it will contain £1 million. If you take both, it will be empty. And if I am wrong, I will give £1 million, which you see before you, to a random member of the crowd!'
   Everyone gasped as Marco opened a suitcase full of £50 notes.
   'Ladies and gentlemen. I have performed this miracle one hundred times and never been wrong, as independent observers have testified. And if you observe the closed box, which is now ten feet from me, you will see that nothing I do can now alter its contents. So, Frank. What will you choose?'

Source: Newcomb's Paradox, devised by William Newcomb and popularised in 'Newcomb's Problem and Two Principles of Choice' by Robert Nozick, in Essays in Honour of Carl G. Hempel (1970).

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 124.
---------------------------------------------------

Okay... So if you were Frank, what box or boxes would you choose? Why? And what would the outcome actually be? I'll be back on Friday to discuss.

Or will I?!?

0 Comments

An Evolutionary Response to Hume’s Missing Shade of Blue

2/12/2016

0 Comments

The House of Blues museum in Clarksdale, Mississippi.

There's a lot at stake in this week's thought experiment. A lot more, if you'll excuse the pun, than meets the eye...

David Hume introduced this experiment during his effort to fully describe the features of Empiricism. From my Philosophy 101 page:

Empiricism - is a theory of knowledge that asserts that knowledge comes only or primarily via sensory experience. Empiricism emphasizes evidence, especially as discovered in experiments. It is a fundamental part of the scientific method that all hypotheses and theories must be tested against observations of the natural world rather than resting solely on a priori reasoning, intuition, or revelation.

With that in mind—especially the part about knowledge coming only via sensory experience—let's look at the particulars of this week's experiment.

---------------------------------------------------
Imagine living your whole life in a complex of apartments, shops, and offices with no access to the outdoors. That pretty much sums up life for inhabitants of the massive space stations Muddy and Waters.
   The creators of the stations had introduced some interesting design features in order to test our dependence on experience for learning. On Muddy, they ensured that there was nothing sky-blue on the whole of the ship; on Waters, there was nothing blue at all. Even the inhabitants were chosen so that none carried the recessive gene responsible for blue eyes. To avoid anything blue being seen (such as veins) the lighting in the station was such that blue was never reflected, so veins actually appeared black.
   When those born on the stations reached eighteen, they would be tested. Those on Muddy would be shown a chart with all the shades of blue, with sky-blue missing. The subjects would be asked if they could imagine what the missing shade looked like. They would then be shown a sample of the color and asked if this is what they had imagined.
   Those on Waters would be asked if they could imagine a new color, and then if they could imagine what color needs to be added to yellow to produce green. They too would then be shown a sample and asked if they had imagined that. The results would be intriguing...

Source: Book two of An Essay (sic) Concerning Human Understanding by David Hume (1748)

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 121.
---------------------------------------------------

It's funny that Baggini (or his publishers) made a mistake in the source reference here, citing the title of John Locke's Essay Concerning Human Understanding when wikipedia explicitly points out that "it should not to be confused with" David Hume's Enquiry Concerning Human Understanding, but nonetheless Baggini adds an intriguing step to the original experiment, which I'll get to a bit later. First, I must tackle what Hume wrote, which has come to be known as The Missing Shade of Blue. He argued "that all perceptions of the mind can be classed as either 'Impressions' or 'Ideas'. He further argues that:

"We shall always find, that every idea which we examine is copied from a similar impression. Those who would assert, that this position is not universally true nor without exception, have only one, and at that an easy method of refuting it; by producing that idea, which, in their opinion, is not derived from this source."

Just two paragraphs later though, Hume seems to provide just such a destructive idea that arises without a sense impression. He says:

"There is, however, one contradictory phenomenon, which may prove, that it is not absolutely impossible for ideas to arise, independent of their correspondent impressions. I believe it will readily be allowed that the several distinct ideas of colour, which enter by the eye...are really different from each other; though, at the same time, resembling. Now if this be true of different colours, it must be no less so of the different shades of the same colour; and each shade produces a distinct idea, independent of the rest. ... Suppose, therefore, a person to have enjoyed his sight for thirty years, and to have become perfectly acquainted with colours of all kinds, except one particular shade of blue, for instance, which it never has been his fortune to meet with. Let all the different shades of that colour, except that single one, be placed before him, descending gradually from the deepest to the lightest; it is plain, that he will perceive a blank, where that shade is wanting, and will be sensible, that there is a greater distance in that place between the contiguous colours than in any other. Now I ask, whether it be possible for him, from his own imagination, to supply this deficiency, and raise up to himself the idea of that particular shade, though it had never been conveyed to him by his senses? I believe there are few but will be of opinion that he can: And this may serve as a proof, that the simple ideas are not always, in every instance, derived from the correspondent impressions; though this instance is so singular, that it is scarcely worth our observing, and does not merit, that for it alone we should alter our general maxim."

So, despite Hume's uncharacteristic dismissal of such a singular instance, "scarcely worth observing," this stubborn little problem seems to undermine the whole underpinnings of empiricism and physicalism. And that's a really big deal!

Surprisingly, this has remained a stubborn problem. On wikipedia, there are currently six ways listed that people have attempted to deal with it, all of which suffer from problems.

There is no problem - Hume was wrong when he claimed that it was possible to form an idea of the missing shade. (This is clearly wrong as new shades can be imagined and invented all the time. Think of recent introductions of high-viz products.)
Mental mixing - just as paint cans can be mixed in a store, our brain can mix the idea of colours. (This is clearly wrong as we can't deduce things like blue + yellow = green.)
Colours as complex ideas - colours are already mixed up so we can imagine their components like we can imagine the different properties of an apple = crunchy + juicy + red + sweet, etc. (This is mostly wrong as you can't deduce yellow = green - blue.)
It doesn't undermine Hume's main concern - he doesn't claim to be perfect, but his principles are still useful for metaphysical debates. (This is clearly wrong as Hume himself states that any single instance of an idea from outside a sense impression would admit dualism, rationalism, or idealism into any argument.)
The exception really is singular - colours and their shades can be organised together in a way that other things can't be. (This is also wrong because any singular example can be extended to others, which collectively undermine the general claim that ideas depend on impressions.)
Hume needs an exception - he later makes larger claims that empirical discoveries are only ever probable so he must have found it good to find possible exceptions that prove we aren't certain. (This is nonsense as this particular possibility—imagining things with no basis for getting there—would destroy all the rest of his assumptions.)

As the conclusion to the wikipedia entry states, "None of the suggested solutions are without difficulty." You don't say! Even Massimo Pigliucci tried to tackle this a few years ago in a blog post and just ended up saying Hume found a "hypothetical" hole in his argument. Big deal. Pragmatically it doesn't matter so "philosophers should just relax about it." What an embarrassment! Hume had no access to modern scientific knowledge so he can be forgiven, but Pigliucci, who has PhD's in philosophy AND biology, should have seen right through all this. For us to do so, we'll need a brief detour into the way our eyes function and see colour.

Over on science presenter Steve Mould's blog, he made a really nice post about our eyes that tells us what we need to know, so I'll just quote that at length:

"At the back of our eyes there are little cones that collect light. When light falls on one of these cones it sends a message to the brain to let it know. The more light that falls, the stronger the signal that is sent to the brain. But what about colour? It turns out that there are three types of cone that correspond to red, green and blue. So if you're looking at something blue, it's the blue-sensitive cones that react and tell the brain there's something blue out there and so on. What happens when you look at something yellow? Yellow is inbetween red and green on the spectrum. Because it's not quite red and not quite green it excites both the red-sensitive cones and green-sensitive cones but only a bit! The brain gets a signal from both cones and deduces that it must be looking at something in the middle. Something yellow! Correct."

A couple of pictures help add some clarity to this. Here is a picture of the colour spectrum flipped around to (sort of) line up with a chart of the overlapping spectral sensitivities of our three cones as plotted against wavelengths of light:

So, now that we've got a consilient view of the problem across the disciplines of biology and philosophy (ahem, Massimo), we understand how we can imagine particular shades of blue even if we haven't seen them yet. Physically, it's simply a matter of how excited our blue cones have been in the past. We may not be able to "know" what peaks on those cones might look like without seeing them, but we can easily imagine points in between levels of excitement we have seen. This is simply analogous to imagining what a 5 kg weight dropped on my toe would feel like once I have had a 2 kg and 10 kg weight dropped on it. We can fill in the gaps rather easily. Similarly, I might not "know" what a 200 kg weight dropped on my toe would feel like, but I could roughly extend my imagination to it once I have some experience in the matter.

This answers Hume's missing shade of blue, and it answers Baggini's experiment on the space station Muddy where only sky-blue was missing. But what about on Waters where no blue has ever been seen?

This is akin to Mary's Knowledge Problem, which I answered in my reply to thought experiment 13. In that thought experiment, Mary "knows everything" there is to know about red but has never seen red. Once she does, she learns something new, which supposedly shows there must be something beyond the physical world. As I said, this is hogwash "because Mary does not have 'all the physical information' and cannot know 'all there is to know' about this subject without having experienced it firsthand. Why? Precisely because we live in a physical universe where mental imaginings are not enough to move the physical atoms that make up the nerves in our eyes and the synapses in our brains. In philosophical terms, there is a real epistemic barrier to what we can learn no matter how much we sit in our rooms and read and think."

Looking at the colour charts above, we see that in Mary's case, her red cone has never fired so she cannot imagine what that sense organ feels like. On the spaceship Waters, it would be the blue cone that had never fired. This is a difference in kind, rather than a difference in degree, as compared to the case of the missing shade of blue. Mary, or the people on Waters, would feel the same impotence as we do when we try to imagine what birds can see with their fourth cone, which enables them to see ultraviolet light and magnetic fields. (How cool is bird vision!)

That would seem to be that, but In Baggini's writeup to this thought experiment, he raised another intriguing question that is worth examining. He said:

"Even if those born on Waters could imagine blue, that still leaves one question unanswered. Can they do this because, as humans, they are born with some kind of innate sensitivity to blue, or could they imagine any colour?"

As I said, the fact that their blue cones have never been activated would leave the people on Waters unable to imagine blue, but in Richard Dawkins' latest memoir Brief Candle in the Dark, he made an interesting point about our innate sensitivity to things, which, even if unfelt to date, could be discovered to exist, but only for colours our ancestors have experienced (barring the existence of a recent or dormant mutation). He said:

"There is a sense in which the DNA of a species could, in principle, be read out as a kind of description of the way of life at which the species excels. I have mentioned this idea of 'the Genetic Book of the Dead' in several of my book, but I argued it most fully in the chapter of that name in Unweaving the Rainbow. Here's one of the ways I introduced it:

' ... If you find an animal's body, a new species previously unknown to science, a knowledgeable zoologist allowed to examine and dissect its every detail should be able to 'read' its body and tell you what kind of environment its ancestors inhabited: desert, rain forest, arctic tundra, temperate woodland, or coral reef. The zoologist should be able to tell you, by reading its teeth and guts, what it fed on. Flat, millstone teeth and long intestines with complicated blind alleys indicate it was a herbivore; sharp, shearing teeth and short, uncomplicated guts indicate a carnivore. The animal's feet, and its eyes and other sense organs spell out the way it moved and how it found its food. Its stripes or flashes, its horns, antlers, or crests provide a readout, for the knowledgable, of its social and sex life." (my emphasis added)

Fascinating. And with that, I'll finish up with a short video of another fascinating colour fact I learned about while writing this. It's about the curious case of magenta. That's a colour that is not found in the visible spectrum of light, yet we all see it, and it's actually rather common in nature. Intrigued? Watch this 5-minute video from the Royal Institute.

This might have made a much more interesting example for Hume had he been aware of this, but magenta is still created in our physical brains when two cones are activated—the blue and red cones at each end of the visible spectrum—while our third one in the middle is not activated, so physicalism and empiricism still hold.

It's always surprising to me to find philosophical problems like this that haven't been solved yet when it seems they easily could have been with the benefits of an evolutionary science perspective. But maybe that's to be expected from thinkers who haven't had the right parts of their brain tickled yet. Next!

0 Comments

Thought Experiment 41: Getting the Blues

2/8/2016

3 Comments

Is Iceland's Blue Lagoon really blue?

How much does experience shape our knowledge? Entirely? Or are there some exceptions of things we can just "know"?

---------------------------------------------------
Imagine living your whole life in a complex of apartments, shops, and offices with no access to the outdoors. That pretty much sums up life for inhabitants of the massive space stations Muddy and Waters.
   The creators of the stations had introduced some interesting design features in order to test our dependence on experience for learning. On Muddy, they ensured that there was nothing sky-blue on the whole of the ship; on Waters, there was nothing blue at all. Even the inhabitants were chosen so that none carried the recessive gene responsible for blue eyes. To avoid anything blue being seen (such as veins) the lighting in the station was such that blue was never reflected, so veins actually appeared black.
   When those born on the stations reached eighteen, they would be tested. Those on Muddy wuld be shown a chart with all the shades of blue, with sky-blue missing. The subjects would be asked if they could imagine what the missing shade looked like. They would then be shown a sample of the colour and asked if this is what they had imagined.
   Those on Waters would be asked if they could imagine a new colour, and then if they could imagine what colour needs to be added to yellow to produce green. They too would then be shown a sample and asked if they had imagined that. The results would be intriguing...

Source: Book two of An Essay Concerning Human Understanding by David Hume (1748)

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 121.
---------------------------------------------------

What do you think? Could either inhabitants imagine their missing colour before ever seeing it? Does it make a difference if they are trying to imagine a new shade or a new colour? I'll be back on Friday with my evolutionary perspective on this.

3 Comments

Response to Thought Experiment 40: The Rocking-Horse Winner

2/5/2016

0 Comments

A black swan! Who saw that coming?

This week's thought experiment seems pretty easy on the face of it, but it does bring up a thorny issue—the problem of gaining knowledge through induction. As the philosopher C. D. Broad said, "induction is the glory of science and the scandal of philosophy." Let's look at the thought experiment and then discuss why this is the case.

---------------------------------------------------
Paul knew which horse would win the Derby. At least, he felt certain he knew, and when he had felt this certainty in the past, he had never been wrong.
Paul's conviction was not based on studying the horses' form. Nor could he see the future unfolding in a vision. Rather, the name of the winning horse would just come to him, as he rode back and forth on his rocking horse, which he had really outgrown.
It was not that Paul won all his bets (or those made on his behalf by the adults who shared his secret). Sometimes he was less sure, and on other occasions he didn't really know at all and just guessed. But he never bet a large amount in those circumstances. When he was completely sure, however, he put down almost all the money he had. The method had never let him down yet.
Oscar, one of his adult collaborators, had no doubt that Paul possessed an uncanny ability, but he was not sure that Paul really knew the winners. It wasn't enough that Paul had always won so far. Unless he knew why he had got it right, the foundations of his belief were far too shaky to hold true knowledge. However, that did not stop Oscar from betting some of his own money on Paul's tips.

Sources: 'The Rocking-Hourse Winner' by D.H. Lawrence (1926); lectures by Michael Proudfoot

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 118.
---------------------------------------------------

As Plato defined it, knowledge is justified true belief. But how has our young horserace handicapper come by his own belief in his "knowledge"? A few lucky bets? Some strong feelings? Is that justified? For the rest of us, we've seen far too many instances of betting at the tracks to know that predictions by bettors are rarely right more than at levels of random chance (once odds are accounted for). We don't see people go on miraculous winning streaks that prove they are somehow much better at this than everyone else. So we think Paul is being silly and it's only a matter of time before one of his big bets on a "sure thing" goes wrong and he goes bust.

In both Paul's case and in our own, however, everyone is relying on inductive reasoning for their beliefs. The Oxford English Dictionary defines induction as: the process of inferring a general law or principle from the observation of particular instances. Paul has seen his method work in the past; he thinks the general law is that it will work in the future. We've seen bettors fail in the past; we think they'll all fail in the future. Who's to say who's right? As the Stanford Encyclopedia of Philosophy entry on the induction problem says, "What distinguishes good from bad inductions?"

It turns out there's not a great answer to this question as induction has never been fully justified. The Ancient Greek skeptic Sextus Empiricus was possibly the first to question the validity of inductive reasoning when he wrote:

"When they propose to establish the universal from the particulars by means of induction, they will effect this by a review of either all or some of the particulars. But if they review some, the induction will be insecure, since some of the particulars omitted in the induction may contravene the universal; while if they are to review all, they will be toiling at the impossible, since the particulars are infinite and indefinite."

That's the problem of induction known as generalising—i.e., trying to define "the properties of a class of objects based on some number of observations of particular instances of that class (for example, the inference that "all swans we have seen are white, and, therefore, all swans are white", before the discovery of black swans)." But there is a second common objection to induction as well.

David Hume is the philosopher who is famously attached to this part of the induction problem. He said there was no justification for "presupposing that a sequence of events in the future will occur as it always has in the past (for example, that the laws of physics will hold as they have always been observed to hold)." Hume called this the principle of "uniformity of nature", but to rely on it by simply pointing to past examples of the uniformity of nature was merely begging the question in a vicious circularity.

For practical purposes, people just accept this and rely on the uniformity of nature until proven otherwise. So far, that has worked. But really, philosophers know we cannot Know this will always be the case.

When talking about this issue though, it's important to point out how philosopher Karl Popper proposed we can get around it. He held that:

"Induction has no place in the logic of science. Science in his view is a deductive process in which scientists formulate hypotheses and theories that they test by deriving particular observable consequences. Theories are not confirmed or verified. ... The best we can say of a hypothesis is that up to now it has been able to show its worth, and that it has been more successful than other hypotheses. ... This appraisal of the hypothesis relies solely upon deductive consequences (predictions), which may be drawn from the hypothesis: There is no need even to mention “induction”."

Popper said this meant the scientific method relies on falsifiability, or the liability of a theory to counterexample. Highly falsifiable theories thus make stronger assertions and are in general more informative.

So we may use the iterative nature of the scientific method to tease out causes, inventing new and different experiments that rule out other causes until we are "sure" we have isolated the true cause. At least to the best of our present abilities. And it must be said that we've been using this method to improve the scientific method as well—gradually emphasising repeatability, independent observations, falsifiability, double blind methodology, etc.—all the time trying to correct for the fallacies our senses and reason are susceptible to. But as I've pointed out several times on this blog, this all means our knowledge is only ever probabilistic so we must act with confidence and caution appropriate to the probability, being especially careful in realms where knowledge is uncertain and consequences of error are large.

So, it looks like Paul's belief that he can pick the horsies is a theory that isn't justified and has generally been falsified by other gamblers in the past. I, therefore, wouldn't put my own money behind him. But then again, we aren't really told how these winners "come to him" while he's on his hobby horse so maybe there's a hidden cause there that is new to us. I guess we'll just have to wait and see...

0 Comments

Thought Experiment 40: The Rocking-Horse Winner

2/2/2016

0 Comments

Hey, that's Novak Djokovic! He's a safe bet, right?

After a very long and difficult thought experiment last week, it sure would be nice to get something a bit easier to tackle. Good thing I woke up feeling sure that will be the case. Let's see if I'm right.

---------------------------------------------------
Paul knew which horse would win the Derby. At least, he felt certain he knew, and when he had felt this certainty in the past, he had never been wrong.
Paul's conviction was not based on studying the horses' form. Nor could he see the future unfolding in a vision. Rather, the name of the winning horse would just come to him, as he rode back and forth on his rocking horse, which he had really outgrown.
It was not that Paul won all his bets (or those made on his behalf by the adults who shared his secret). Sometimes he was less sure, and on other occasions he didn't really know at all and just guessed. But he never bet a large amount in those circumstances. When he was completely sure, however, he put down almost all the money he had. The method had never let him down yet.
Oscar, one of his adult collaborators, had no doubt that Paul possessed an uncanny ability, but he was not sure that Paul really knew the winners. It wasn't enough that Paul had always won so far. Unless he knew why he had got it right, the foundations of his belief were far too shaky to hold true knowledge. However, that did not stop Oscar from betting some of his own money on Paul's tips.

Sources: 'The Rocking-Hourse Winner' by D.H. Lawrence (1926); lectures by Michael Proudfoot

Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 118.
---------------------------------------------------

Of course I was just kidding about having that feeling about this thought experiment being an easy one. But it looks like I might be right anyway. What do you think? Have you ever inexplicably known something? I'll be back on Friday to discuss. But you already knew that didn't you?

0 Comments

Thought Experiment 44: Till Death Us Do Part

Response to Thought Experiment 43: Future Shock

Thought Experiment 43: Future Shock

Response to Thought Experiment 42: Take the Money and Run

Thought Experiment 42: Take the Money and Run

An Evolutionary Response to Hume’s Missing Shade of Blue

Thought Experiment 41: Getting the Blues

Response to Thought Experiment 40: The Rocking-Horse Winner

Thought Experiment 40: The Rocking-Horse Winner

Subscribe to Help Shape This Evolution

Blog Philosophy

Archives