---------------------------------------------------
'Marco the Magnificent will now demonstrate his extraordinary powers of precognition! You, sir! What is your name?'
'Frank,' replied Frank, to the fairground showman.
'Frank, I know your future! I know all futures, including those of stocks and shares! Which is why I have the money to give away to you in this demonstration of my powers! Behold, two boxes! One you can see is open. It contains £1,000. The other is closed. It contains either £1 million or nothing at all! You may take either box or both. But be warned! I know how you will choose. If you take just the closed box, it will contain £1 million. If you take both, it will be empty. And if I am wrong, I will give £1 million, which you see before you, to a random member of the crowd!'
Everyone gasped as Marco opened a suitcase full of £50 notes.
'Ladies and gentlemen. I have performed this miracle one hundred times and never been wrong, as independent observers have testified. And if you observe the closed box, which is now ten feet from me, you will see that nothing I do can now alter its contents. So, Frank. What will you choose?'
Source: Newcomb's Paradox, devised by William Newcomb and popularised in 'Newcomb's Problem and Two Principles of Choice' by Robert Nozick, in Essays in Honour of Carl G. Hempel (1970).
Baggini, J., The Pig That Wants to Be Eaten, 2005, p. 124.
---------------------------------------------------
Why is this a paradox exactly? In case it wasn't clear from your first read through the problem, here's Baggini's explanation of the difficulty:
"It seems clear that [Frank] should choose only the closed box. That way he will get £1 million, instead of just £1,000. But wait. As Frank reaches for the closed box, a thought enters his mind. That box contains £1 million or it doesn't. Nothing he does can change that fact. So if it does contain the money, it is not going to disappear if he also takes the open box. Similarly, if it is empty, £1 million is not going to magically appear in it if he leaves the open box behind. His choice cannot change what is in the closed box. So if he takes the open box or not, the amount in the closed one will remain the same. Therefore he may as well take both, since he can't have less money as a result. Hence we have a paradox. Two lines of reasoning, both seemingly impeccable, lead to contradictory conclusions. One concludes that he should take only the closed box; the other that he may as well take both."
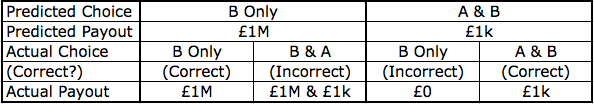
When this problem—known as Newcomb's Paradox—has been discussed in the past, there haven't been any penalties attached for getting the prediction wrong. There were just various versions of who the predictor was (psychic, alien, god, brain scanning computer, etc.) and how certain this predictor was of getting his/her/its prediction right. Without the penalty attached, the permutations for analysis look like this:
So, at the beginning of these initial rules, the clear choice, as Baggini points out, is "B only". If Marco predicts that, Frank's payout is £1,000,000. Once that prediction is set though, then "A & B" becomes the best choice for Frank as his payout would then climb to £1,001,000. Marco would know this, however, so he would predict "A & B", which would drop Frank's payout to £1,000. Frank would know this is worse than where he started though, so he would revert to "B only", which Marco would predict, and we'd be back to the beginning. We're stuck in a loop here because for every choice Frank makes, he can see how it would be better to flip that choice, but since Marco can always foresee that, he keeps flipping his choice as well. Rather than stand there forever without being able to make a decision (which yields £0 while you are waiting), Frank might as well take the perfection of the predictions at face value and choose "B Only" even if £1,000,000 is less than £1,001,000.
Traditionally for this problem, there is also some small doubt expressed about how perfect these predictions are. In that case, you also have to weigh the chance of getting £0 into the mix in the event that your choice of "B Only" was incorrectly predicted. In those variations of this problem, it becomes a question of how certain you need to be of getting £1,000,000 vs. the absolute certainty of getting £1,000. There's some element of that here since we're dealing with a carnival barker backed by "independent observers", but it's no use speculating over the trustworthiness of this scenario as it's really unknowable. The mathematical solution in this case would be to take "B Only" for any certainty over 1% about the accuracy of the prediction (then the expected value of "B Only" > £1M x 1% > £1,000), but as I said, this quantification of certainty is really unknowable.
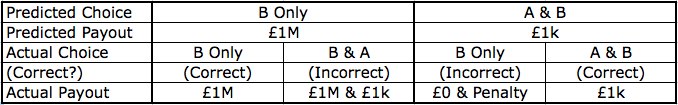
Now, let's look at what happens given the wrinkle Baggini has added about the £1,000,000 penalty being paid to a random member of the crowd in the event that Marco's prediction is wrong. Plugging that change into the above table, we see:
This logic problem has dragged on farther than I wanted it to, but I hope it has been rigorously explained enough. I could talk about the epistemology of not being able to change the past with a present choice. I could talk about the effect of free will on efforts to predict human behaviour. I could talk about the realm of emotional vs. rational decision making about gains, losses, and missed opportunities. Or I could talk about the math behind Frank making various deals with the audience to share the £1M penalty as a hedge against the downside of choosing "B Only". But by now I hope that you could predict what I would say about any one of those discussions....